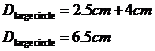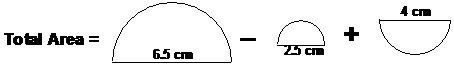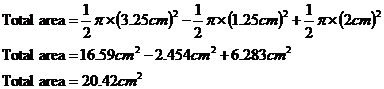Areas of composite shapes
Composite shapes are shapes that are made up of other shapes. For instance, you could make a house-like shape by using a square and a triangle, like this:
Sponsored Links
There is a general approach you can use to solve for the areas of these types of shapes:
· Draw a diagram of the shape and label all the information you know about it – side lengths, any areas of it you already know etc…
· Split the figure up into regular shapes which you know area formulas for, and then write a shape equation saying how to get the final shape from these regular shapes.
· Pick the appropriate area formula for each regular shape in your equation, and use it to work out the areas of each of them. Then add these areas together and solve the shape equation.
|
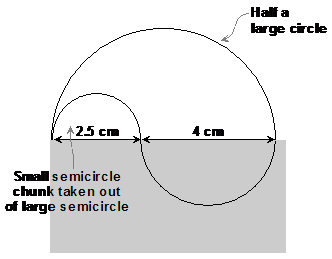
Find the area of the following shape:
|
|
Solution |
|
The first time I ever came across this problem I was bamboozled. I knew you had to be able to break it up into circles, but I couldn’t work out at first how to do it. Practice with these questions helps a lot – as you do more questions you ‘see’ more quickly how to split these composite shapes up into regular shapes. Step 1 is already done for us – there’s a diagram with all the information we know on it. Step 2 involves splitting this shape up into regular shapes. One way to do this is to start from the biggest possible shape and work out what bits you need to add or subtract from it:
So we’ve got half of a large circle (a semicircle) in our diagram, but there’s a chunk taken out of it – there’s a much smaller semicircle chunk taken out of it. To write our shape equation we need to know the diameters of the large and small semicircles. We already know the small one – 2.5 cm. The large one we can work out: So we can already write a bit of our shape equation:
This covers the top half of the shape, but we’ve also got an extra mid sized semicircle attached as the bottom half of the composite shape, so we need to add this to our equation to finish it:
Step 3 involves picking the appropriate formula to work
out the areas of each of these regular shapes. They’re all semicircles, so
we can use the where rbig = 3.25 cm, rsmall = 1.25 cm and rmedium = 2 cm. |